
Set 1
Fuel contamination levels at each of 20 petrol pumps P1, P2, …, P20 were recorded as either high, medium, or low.
1. Contamination levels at three pumps among P1 – P5 were recorded as high.
2. P6 was the only pump among P1 – P10 where the contamination level was recorded as low.
3. P7 and P8were the only two consecutively numbered pumps where the same levels of contamination were recorded.
4. High contamination levels were not recorded at any of the pumps P16 – P20.
5. The number of pumps where high contamination levels were recorded was twice the number of pumps where low contamination levels were recorded.
Solution

Explanation:
By point 3, we know that P7 and P8 are recorded at same level. Except these two, no other consecutive pumps have same level of contamination.
By point 2, we know that only P6 is recorded as Low and all other are recorded as either Medium or High.
By point 1, We know that P1 to P5 have 3 high and 2 medium pumps. As we know that the 3 high pumps cannot be consecutive then P1, P3 and P5 must be highly Contaminate and P2 and P4 must be medium.
Next P6 is recorded as Low hence we have two possibilities for P7, P8, P9 and P10 as High, High, Medium, High or Medium, Medium, High, Medium.
In case 1, we have 6 highly contaminated pumps and in case 2 we have 4 highly contaminated pumps.
Point 6 states that Highly contaminated pumps are twice than Lowly contaminated.
The sum of these two must be a multiple of 3.
So there can only be 8 medium rated pumps making 8 high rated and 4 low rated.
In case we assume medium to be 5 and high and low as 10 and 5, the situation will violate the case then there must be every other pump rated as high while P16 to P20 has no high pump.
Now, if we take the two cases stated above, in 2nd case we have 4 highly contaminated pumps. We know there are 8 highly contaminated pumps in total so now P11 to P15 will have to have 4 contaminated pumps which is not possible because no two consecutive pumps can have some level of contamination.
After eliminating case 2, we have first 10 pumps as
| P1 | P2 | P3 | P4 | P5 | P6 | P7 | P8 | P9 | P10 |
| High | Med | High | Med | High | Low | High | High | Med | High |
P16 to P20 have no High contaminated Pumps so the two cases will be M, L, M, L, M or L, M, L, M, L.
In case 1, we have 6 medium rated and 3 Low rated pumps. In case 2, we have 5 medium and 4 (All) low rated pumps.
Nothing much can be said about the rest so we move to the questions.
Question 1
Q.1 Which of the following MUST be true?
1. The contamination level at P13 was recorded as low.
2. The contamination level at P10 was recorded as high.
3. The contamination level at P20 was recorded as medium.
4. The contamination level at P12 was recorded as high.
Answer: we know that P10 is recorded as High. Others may or may not be true. So the answer is option 2.
Question 2
What best can be said about the number of pumps at which the contamination levels were recorded as medium?
1. At most 9
2. More than 4
3. At least 8
4. Exactly 8
Answer: There are 8 medium level contaminated pumps.
Question 3
If the contamination level at P11 was recorded as low, then which of the following MUST be true?
1. The contamination level at P18 was recorded as low.
2. The contamination level at P12 was recorded as high.
3. The contamination level at P15 was recorded as medium.
4. The contamination level at P14 was recorded as medium.
Answer: In this we know that P11 was recorded as low. This is possible in case 1 where P16 to P20 had only 2 Low level contaminated pumps. So they must have been recorded as Med, Low, Med, Low and Med respectively. This eliminates option 1 as P18 is rated as Medium.
Option 3 is eliminated because P16 is recorded as medium and so P15 must be High (Cant be low as all the low recorded pumps have found already).
But here we see that if P15 is recorded as Highly contaminated pump then P14 can only be Medium contaminated. So the answer is option 4.
Question 4
If contamination level at P15 was recorded as medium, then which of the following MUST be FALSE?
1. Contamination levels at P10 and P14 were recorded as the same.
2. Contamination levels at P11 and P16 were recorded as the same.
3. Contamination level at P14 was recorded to be higher than that at P15.
4. Contamination levels at P13 and P17 were recorded as the same.
Answer: This gives us that P15 is rated as Medium and so P16 will be rated as low and our case 2 will be applicable here that is no Low recorded contamination in P11 to P15.
If P15 is medium so P14 must be high. P10, as we already know, is high. Option 1 is true hence not our answer.
For the same reason as above, Option 3 is true hence not our answer.
P17 is Medium contaminated pump and P13 can also be Medium contaminated so not necessarily false.
P11 must beMedium because P10 is High and P16, as per our case, is Low. This must befalse.
Set 2
An ATM dispenses exactly Rs. 5000 per withdrawal using 100, 200 and 500 rupee notes. The ATM requires every customer to give her preference for one of the three denominations of notes. It then dispenses notes such that the number of notes of the customer’s preferred denomination exceeds the total number of notes of other denominations dispensed to her.

Explanation:
Question 1
In how many different ways can the ATM serve a customer who gives 500 rupee notes as her preference?
Answer: we can take 100x + 200y + 500z = 5000 where x, y and z are the number of notes.
Or say, x + 2y + 5z = 50.
Now the condition we have here is x + y < z
If z=10, only 1 case as 5*10 = 50.
If z=9, we get x + 2y + 5*9 = 50 => x + 2y = 5. This can be done in 3 ways at x=1, 3 and 5. All these values will be less than z.
If z=8, we get x + 2y + 5*8 = 50 => x + 2y = 10. This can be done at x = 0, 2, 4, 6, 8 and 10.
But in this case, x + y < 8 is valid only at x = 0, 2 and 4 so 3 cases.
Total possible cases are 7.
Question 2
If the ATM could serve only 10 customers with a stock of fifty 500 rupee notes and a sufficient number of notes of other denominations, what is the maximum number of customers among these 10 who could have given 500 rupee notes as their preferences?
Answer: To maximize the number of people getting 500 rupee notes, we need to minimize the 500 rupee notes in every transaction. As seen in the previous question, a person giving 500 rupee note as his preference will receive at least 8 500 rupee notes. So if everyone receives 8 500 rupee notes then maximum number of people who can get these 50 notes is 50 / 8 = 6.25.
Maximum number of people who give 500 as their preference are 6.
Question 3
What is the maximum number of customers that the ATM can serve with a stock of fifty 500rupee notes and a sufficient number of notes of other denominations, if all the customers are to be served with at most 20 notes per withdrawal?
- 10
- 13
- 12
- 16
Answer: we only have a limit on 500 rupee notes so we will be using a maximum of 200 rupee notes.
By using 200 rupee notes only, we need 25 notes to make 5000, 20 notes to make 4000 and 15 notes to make 3000.
We can make 5,000 rupees with 15 200 rupee notes and 4 500 rupee notes, that is, with a total of 19 notes.
Now we are using 4 500 rupee notes in every transaction and we have 50 in total so the maximum number of customers that can be served like this are 50 / 4 = 12.5 that is 12 customers.
Question 4
What is the number of 500 rupee notes required to serve 50 customers with 500 rupee notes as their preferences and another 50 customers with 100 rupee notes as their preferences, if the total number of notes to be dispensed is the smallest possible?
- 800
- 750
- 900
- 1400
Answer: We have to minimize the number of notes required so we maximize the usage of 500 rupee notes.
For customers having 500 rupee note as their preference, we can give them 10 500 rupee notes each making total requirement of 500 rupee notes here as 50 * 10 = 500.
For customers having 100 rupee note as their preference, they can be given 10 100 rupee notes and 8 500 rupee notes for our purpose. The requirement of 500 rupee notes, in this case becomes 50 * 8 = 400.
Total requirement = 500 + 400 = 900.
Set 3
The multi-layered pie-chart below shows the sales of LED television sets for a big retail electronics outlet during 2016 and 2017. The outer layer shows the monthly sales during this period, with each label showing the month followed by sales figure of that month. For some months, the sales figures are not given in the chart. The middle-layer shows quarter- wise aggregate sales figures (in some cases, aggregate quarter-wise sales numbers are not given next to the quarter). The innermost layer shows annual sales. It is known that the sales figures during the three months of the second quarter (April, May, June) of2016 form an arithmetic progression, as do the three monthly sales figures in the fourth quarter (October, November, December) of that year.
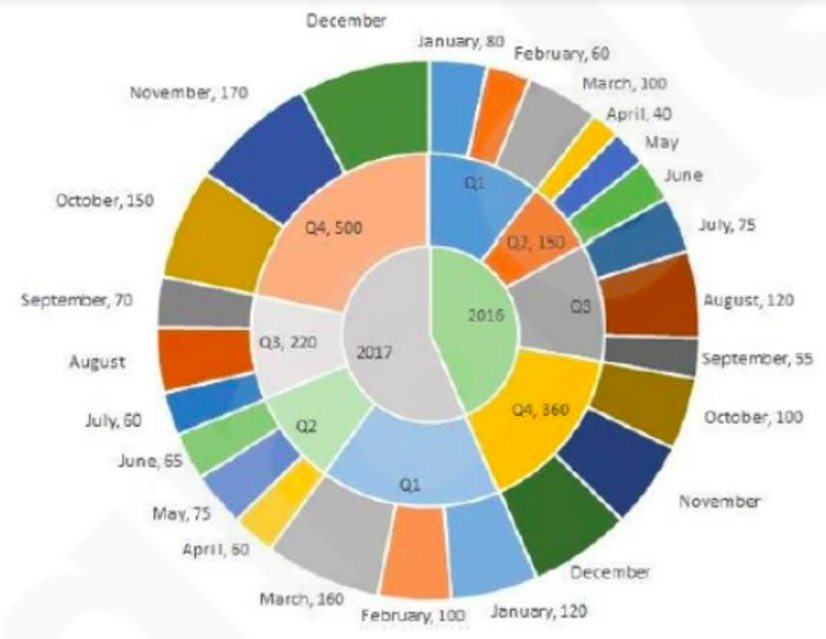
Solution

Explanation:
In this, we have been given sales of each months (except some), sum of 3 months of each quarter (except some).
Thing to do here is that we have to do the sum of the months of each quarter and find the missing values.
In quarter 1 of 2016, sum of the quarter will be 80 + 60 + 100 = 240
In quarter 2 of 2016, sum of the quarter is 180. In the set, it is also given that sale of April, May and June is in AP. So the revenue of April, May and June will be 40, 60 and 80 respectively.
In quarter 3 of 2016, sale of July, August and September is 75, 120 and 55 respectively. Then the sum of the quarter will be 250
Similarly, we keep on finding the values of each month and quarter.
The final table for this will be
| Year | Month | Monthly Sale | Quarterly Sale | Yearly Sale |
| 2016 | January | 80 | 240 | 1030 |
| February | 60 | |||
| March | 100 | |||
| April | 40 | 180 | ||
| May | 60 | |||
| June | 80 | |||
| July | 75 | 250 | ||
| August | 120 | |||
| September | 55 | |||
| October | 100 | 360 | ||
| November | 120 | |||
| December | 140 | |||
| 2017 | January | 120 | 380 | 1300 |
| February | 100 | |||
| March | 160 | |||
| April | 60 | 200 | ||
| May | 75 | |||
| June | 65 | |||
| July | 60 | 220 | ||
| August | 90 | |||
| September | 70 | |||
| October | 150 | 500 | ||
| November | 170 | |||
| December | 180 |
Question 1
What is the percentage increase in sales in December 2017 as compared to the sales inDecember 2016?
- 22.22
- 28.57
- 50.00
- 38.46
Answer: Sales in December 2017 increased from December 2016 that is became 180 from 140.
This increase is 28.57%.
Question 2
In which quarter of 2017 was the percentage increase in sales from the same quarter of 2016 the highest?
- Q3
- Q1
- Q4
- Q2
Answer: By the table, percentage increase in quarterly sales was 58.33% for the first quarter which was highest. The answer is option 2, quarter 1.
Question 3
During which quarter was the percentage decrease in sales from the previous quarter’s sales the highest?
1. Q2 of 2016
2. Q2 of 2017
3. Q4 of 2017
4. Q1 of 2017
Answer: Sale decrease percent in Quarter 2 in 2017 was highest in Quarter 2 of 2017 which is47.37%.
Question 4
During which month was the percentage increase in sales from the previous month’s sales the highest?
1. October of 2016
2. October of 2017
3. March of 2016
4. March of 2017
Answer:Percentage increase in sales compared to previous month is Highest in October2017 that is 114.29%.
Set 4
1600 satellites were sent up by a country for several purposes. The purposes are classified as broadcasting (B),communication (C), surveillance (S), and others (O). A satellite can serve multiple purposes; however a satellite serving either B, or C, or S does not serve O. The following facts are known about the satellites:
1. The numbers of satellites serving B, C,and S (though may be not exclusively) are in the ratio 2:1:1.
2. The number of satellites serving all three of B, C, and S is 100.
3. The number of satellites exclusively serving C is the same as the number of satellites exclusively serving S. This number is 30% of the number of satellites exclusively serving B.
4. The number of satellites serving O is the same as the number of satellites serving both C and S but not B.
Solution

Explanation:
In this, we have a satellite that can serve 4 purposes, one or more than one at a time. So this set looks like a 4 set venn diagram question but as we read, no satellite serving B, C or S, can serve purpose O. So we can make a 3 set venn with external part representing O.
By point 3, we have satellites serving exclusively C and satellites serving exclusively S are equal and each of these numbers is 30% of exclusively B.
So we take Exclusively B as 10a and exclusively C and exclusively S as 3a each which is 30% of 10a.
By point 2, we have all three as 100.
By point 4, we have satellites serving Oare equal to satellite serving both C and S but not B. So we take both of thesevalues as ‘b’.
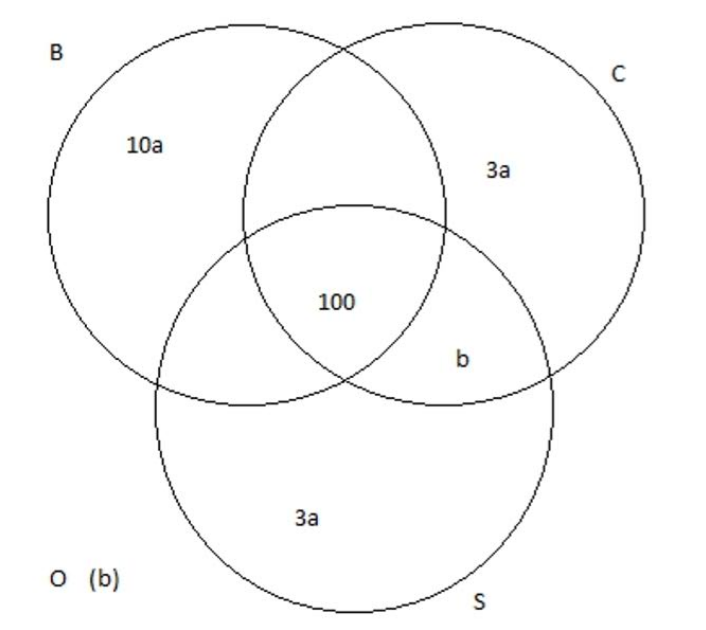
By point 1, satellites serving B are twice the number satellites serving C as well as satellites serving S. And satellites serving C and S are equal.
As total of C and S are equal and the three values that we know of them are also equal (3a, b, 100) then the 4th value that is satellites serving B and C but not S and satellites serving B and S but not C must also be equal. We write them as ‘c’.
Now, we also know that total number of satellites serving B is twice than total number of satellites serving C (or S).
If we equate them, we get
10a + 2c + 100 = 6a + 2b + 2c + 200
Solving, we get
4a – 100 = 2b or
2a – 50 = b.
Now we put the value of b in the venn diagram we have.
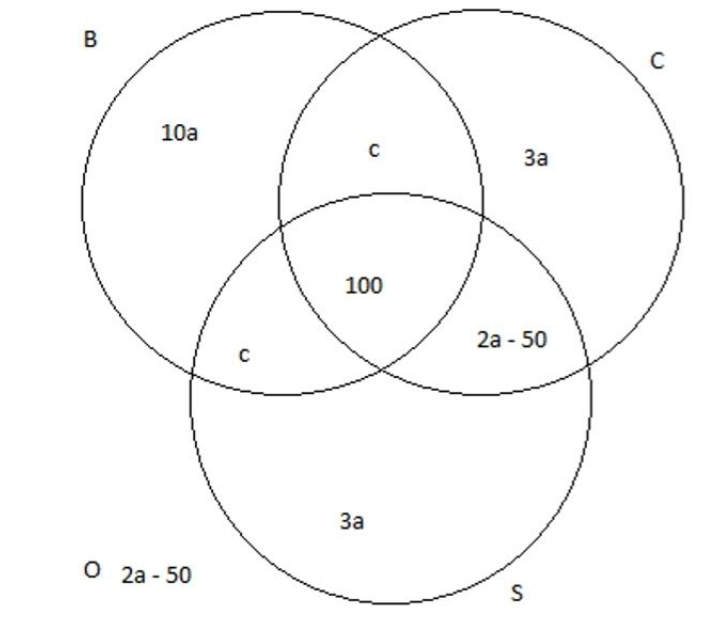
We have all the values in terms of a and c so we can do here is
Sum of all of them is equal to 1600 as total number of satellites in 1600. We get
10a + c + c + 100 + 3a + 2a – 50 + 3a + 2a – 50 = 1600
That is 20a + 2c = 1600 or,
10a + c = 800.
Using this and using b = 2a – 50, we get the minimum and maximum values of a as 25 and 80 respectively.
Consequently, the minimum and maximum value of c becomes 0 and 550.
Question 1
What best can be said about the number of satellites serving C?
1. Must be between 450 and 725
2. Cannot be more than 800
3. Must be at least 100
4. Must be between 400 and 800
Answer: satellites serving C is equal to 3a + 2a – 50 + 100 + c that is
5a + c + 50
Putting the minimum and maximum value of a into it, we get the minimum and maximum number of satellites serving C as 450 and 725.
The answer would be option 1.
Question 2
What is the minimum possible number of satellites serving B exclusively?
- 100
- 250
- 500
- 200
Answer: From the venn, number of satellites serving exclusively B is 10a.
As we know that minimum possible value of a is 25, the answer would be 10a = 10 * 25 = 250.
Question 3
If at least 100 of the 1600 satellites we reserving O, what can be said about the number of satellites serving S?
1. At least 475
2. No conclusion is possible based on the given information
3. At most 475
4. Exactly 475
Answer: Satellites serving O are 2a – 50, and as given in the question, this is at least 100.
So, 2a – 50 >= 100 that means a >= 75 i.e. minimum value ‘a’ is revised as 75. As we know 10a + c = 800, Value of c at this point will be 50.
Number of satellites serving S are 3a + 2a + c – 50 + 100, where minimum value of ‘a’ is 75 and maximum is 80.
Minimum and maximum number of satellites serving S would be 450 and 475.
The answer to the question is option 3, at most 475.
Question 4
If the number of satellites serving at least two among B, C, and S is 1200, which of the following MUST be FALSE?
1. All 1600 satellites serve B or C or S
2. The number of satellites serving B exclusively is exactly 250
3. The number of satellites serving C cannot be uniquely determined
4. The number of satellites serving B is more than 1000
Answer: Satellites serving at least two among B, C and S are 1200. From the venn, this number is 2a – 50 + 100 + c + c that is 2a + 2c + 50.
Equating these we get, 2a + 2c + 50 = 1200
- 2a + 2c = 1150
- a + c = 575
As we know from the solution, 10 + c = 800
Subtracting our new equation from the above one we get
9a = 225 => a = 25
That means c = 550
Inputting these values into the venn, we can obtain all the values.
Option 3 is definitely false.
Set 5
Adriana, Bandita, Chitra, and Daisy are four female students, and Amit, Barun, Chetan, and Deb are four male students. Each of them studies in one of three institutes – X, Y, and Z. Each student majors in one subject among Marketing, Operations, and Finance, and minors in a different one among these three subjects. The following facts are known about the eight students:
1. Three students are from X, three are from Y, and the remaining two students, both female, are from Z.
2. Both the male students from Y minor in Finance, while the female student from Y majors in Operations.
3. Only one male student majors in Operations, while three female students minor in Marketing.
4. One female and two male students major in Finance.
5. Adriana and Deb are from the same institute. Daisy and Amit are from the same institute.
6. Barun is from Y and majors in Operations. Chetan is from X and majors in Finance.
7. Daisy minors in Operations.
Solution

Explanation:
By point 1, we know that X and Y has 3 students each while Z has 2 and both of them in Z are females.
By point 2, Y has 2 male and 1 female student and so X must have the remaining 2 male and 1 female student.
| X | Y | Z |
| 2 male and 1 female | 2 male and 1 female | 2 female |
Also, the female in Y is majoring in Operations.
From point 5, Adriana and Deb are from same institute and Daisy and Amit are from same institute. These two pairs have one male and one female combination so they must be from X or Y. So remaining two females must be from Z. Hence, Bandita and Chitra are from Z.
By point 7, we know that Daisy’s minor is Operations. Point 3 states that 3 females have marketing as their minor so they must be Adriana, Bandita and Chitra.
Also, as the female in Y have Operations as Major, she cannot have Operations as minor too. So Daisy must be in X and Adriana in Y.
And so as per point 5, we can know from which institute are Amit and Deb are.
By point 6, we know from which institute Barun and Chetan are. Putting all the data in the table.
The table of the students would be like this now.
| Adriana | Bandita | Chitra | Daisy | Amit | Barun | Chetan | Deb | |
| Institue | Y | Z | Z | X | X | Y | X | Y |
| Major | Oper. | Oper. | Fin. | |||||
| Minor | Mark. | Mark. | Mark. | Oper. |
By point 2, both the male students from Y have minor in finance.
| Adriana | Bandita | Chitra | Daisy | Amit | Barun | Chetan | Deb | |
| Institue | Y | Z | Z | X | X | Y | X | Y |
| Major | Oper. | Oper. | Fin. | |||||
| Minor | Mark. | Mark. | Mark. | Oper. | Fin. | Fin. |
By point 3, only 1 male student majors in operations. This male student is Barun.
Amit and Deb can only major in Marketing or Finance. Deb has minor in finance so he must have major in marketing only.
Also, from point 4, 2 male students major in finance. Barun and Deb cannot be those two so Amit and Chetan are.
| Adriana | Bandita | Chitra | Daisy | Amit | Barun | Chetan | Deb | |
| Institue | Y | Z | Z | X | X | Y | X | Y |
| Major | Oper. | Fin. | Oper. | Fin. | Mark. | |||
| Minor | Mark. | Mark. | Mark. | Oper. | Fin. | Fin. |
Now, all that we know is that out of Bandita, Chitra and Daisy, one person have major in Finance while others can have any of the other two.
Question 1
Who are the students from the institute Z?
1. Chitra and Daisy
2. Adriana and Daisy
3. Adriana and Bandita
4. Bandita and Chitra
Answer: By the table, Bandita and Chitra are in Z. Option 4.
Question 2
Which subject does Deb minor in?
1. Finance
2. Operations
3. Cannot be determined uniquely from the given information
4. Marketing
Answer: By the table, Deb is minor in Finance that is option 1.
Question 3
Which subject does Amit major in?
1. Finance
2. Operations
3. Marketing
4. Cannot be determined uniquely from the given information
Answer: By the table, Amit is major in Finance that is option 1.
Question 4
If Chitra majors in Finance, which subject does Bandita major in?
1. Finance
2. Operations
3. Cannot be determined uniquely from the given information
4. Marketing
Answer: As we know, only one the 4 females in major in finance. Now that we know it is Chitra, Bandita can major in Marketing or Operations. But in the table we see that Bandita is Minor in Marketing so she cannot major in marketing as well.
Bandita must major in Operations. Option 2.
Set 6
Twenty four people are part of three committees which are to look at research, teaching, and administration respectively. No two committees have any member in common. No two committees are of the same size. Each committee has three types of people: bureaucrats, educationalists, and politicians, with at least one from each of the three types in each committee. The following facts are also known about the committees:
1. The numbers of bureaucrats in the research and teaching committees are equal, while the number of bureaucrats in the research committee is 75% of the number of bureaucrats in the administration committee.
2. The number of educationalists in the teaching committee is less than the number of educationalists in the research committee. The number of educationalists in the research committee is the average of the numbers of educationalists in the other two committees.
3. 60% of the politicians are in the administration committee, and 20% are in the teaching committee.
Solution

Explanation:
By point 1, Bureaucrats in Research and Teaching are equal while both of them are 75% of bureaucrats in Administration. We take number of Bureaucrats n Administration as 4a. 75% of 4a is 3a which is the number of bureaucrats in Reasearch and teaching.
Point 2, states that number of educationalist in Research is the average of educationalist in Teacing and Administratioin. That means the numbers are in Arithmatic progression. Further, educationalist in teaching are less than educationalist in research. We take the mean value as b and others as b-x and b+x.
Point 3 states that Politicians in Administration are 60% of total politicians and 20% are in teaching. We take the total number as 5c distribute them as per data.
We put these points into a table.
| Research | Teaching | Administration | Total | |
| Bureaucrats | 3a | 3a | 4a | 10a |
| Educationalist | b | b – x | b + x | 3b |
| Politicians | c | c | 3c | 5c |
| 3a+b+c | 3a+b-x+c | 4a+b+x+3c | 24 |
Now we have 10a + 3b + 5c = 24.
We also know that a, b and c cannot be 0 as given in the set.
So only possible integral values for a, b and c can be 1, 3 and 1.
The new table we have is given below.
Note: we don’t have the value of x so b-x and b+x will have to be taken in cases as per the table.
| Research | Teaching | Administration | Total | |
| Bureaucrats | 3 | 3 | 4 | 10 |
| Educationalist | 3 | 1 or 2 | 5 or 4 | 9 |
| Politicians | 1 | 1 | 3 | 5 |
| 7 | 5 or 6 | 12 or 11 | 24 |
Question 1
Based on the given information, which of the following statements MUST be FALSE?
1. In the administration committee the number of bureaucrats is equal to the number of
Educationalists
2. In the teaching committee the number of educationalists is equal to the number of politicians
3. The size of the research committee is less than the size of the teaching committee
4. The size of the research committee is less than the size of the administration committee
Answer: Going by the options, we can see that option 3 is necessarily false as number of people in research is definitely more than number of people in teaching.
Question 2
What is the number of bureaucrats in the administration committee?
Answer: By the table, the answer is 4.
Question 3
What is the number of educationalists in the research committee?
Answer: By the table, the answer is 3.
Question 4
Which of the following CANNOT be determined uniquely based on the given information?
1. The size of the research committee
2. The total number of bureaucrats in the three committees
3. The size of the teaching committee
4. The total number of educationalists in the three committees
Answer: By the options, we can see that option 3 cannot be determined by the information.
Set 7
You are given an n×n square matrix to be filled with numerals so that no two adjacent cells have the same numeral. Two cells are called adjacent if they touch each other horizontally, vertically or diagonally. So a cell in one of the four corners has three cells adjacent to it, and a cell in the first or last row or column which is not in the corner has five cells adjacent to it. Any other cell has eight cells adjacent to it.
Question 1
What is the minimum number of different numerals needed to fill a 3×3 square matrix?
Answer: the condition we have here is that no two adjacent cells can have same numeral. That is a horizontal pair, a vertical pair and a diagonal pair have no same number.
| 1 | 2 |
| 3 | 4 |
This would turn out as a 2 * 2 matrix with all different numbers (pair is made by 2).
This 2 * 2 matrix can be filled with 4 different numerals is shown in the figure.
| 1 | 2 | 1 |
| 3 | 4 | 3 |
| 1 | 2 | 1 |
If we want to make a 3 * 3 matrix with the condition of no to adjacent pairs having same numeral, we can do it using the above shown 2 * 2 matrix as in the figure.
In this, we have used the same pattern of as of the 2 * 2 matrix in different ways to form this 3 * 3 matrix. This can be done for any n * n matrix where n>2.
Answer to the question is 4.
Question 2
What is the minimum number of different numerals needed to fill a 5×5 square matrix?
| 1 | 2 | 1 | 2 | 1 |
| 3 | 4 | 3 | 4 | 3 |
| 1 | 2 | 1 | 2 | 1 |
| 3 | 4 | 3 | 4 | 3 |
| 1 | 2 | 1 | 2 | 1 |
Answer: As stated in previous question, 5 * 5 matrix can also be made using the same 2 * 2 matrix pattern as shown in the figure.
The answer to this would be 4.
Question 3
Suppose you are allowed to make one mistake, that is, one pair of adjacent cells can have the same numeral. What is the minimum number of different numerals required to fill a 5×5 matrix?
- 4
- 16
- 25
- 9
Answer: If you look into the matrix above, you can see that one mistake is not enough to eliminate all 4’s.
Also, as you check the options, there is no number less than 4 and we already know that 4 is the least number to make a matrix without mistakes so the answer here would also be 4.
Question 4
Suppose that all the cells adjacent to any particular cell must have different numerals. What is the minimum number of different numerals needed to fill a 5×5 square matrix?
Answer: In this, the condition of adjacent cells having different values is changed. Now, none of the cells around a cell cannot have same numeral such that top cell, top right, top left, right, left, bottom left, bottom right and bottom cell, all these cells must have different numerals.
| 1 | 2 | 3 |
| 4 | 5 | 6 |
| 7 | 8 | 9 |
Now instead of a 2 * 2, we need a 3 * 3 matrix such that given in the figure.
Similarly like in previous questions, any matrix of n * n can be formed using multiple matrixes of 3 * 3, given that n>3.
So the answer here would be 9.
Set 8
A company administers a written test comprising of three sections of 20 marks each – Data Interpretation (DI), Written English (WE) and General Awareness (GA), for recruitment. A composite score for a candidate (out of 80) is calculated by doubling her marks in DI and adding it to the sum of her marks in the other two sections. Candidates who score less than 70% marks in two or more sections are disqualified. From among the rest, the four with the highest composite scores are recruited. If four or less candidates qualify, all who qualify are recruited.
Ten candidates appeared for the written test. Their marks in the test are given in the table below. Some marks in the table are missing, but the following facts are known:
1. No two candidates had the same composite score.
2. Ajay was the unique highest scorer in WE.
3. Among the four recruited, Geeta had the lowest composite score.
4. Indu was recruited.
5. Danish, Harini, and Indu had scored the same marks the in GA.
6. Indu and Jatin both scored 100% in exactly one section and Jatin’s composite score was 10 more than Indu’s.
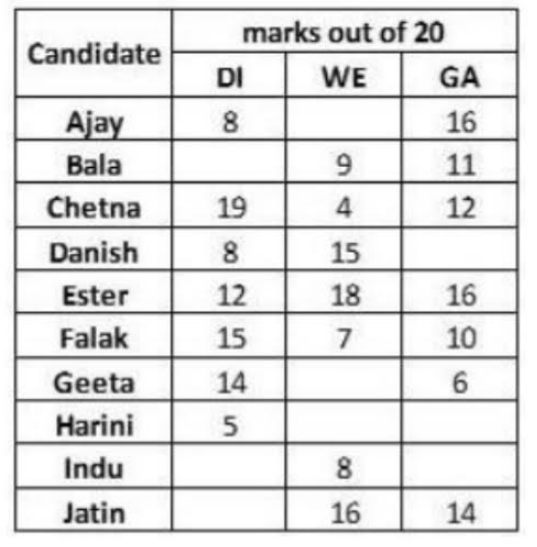
Solution

Explanation:
We make the table with the range of marks these 10 can score.
| Candidate | DI *2 | WE | GA | Composite |
| Ajay | 8 | 0-20 | 16 | 32-52 |
| Bala | 0-20 | 9 | 11 | 20-60 |
| Chetna | 19 | 4 | 12 | 54 |
| Danish | 8 | 15 | 0-20 | 31-51 |
| Ester | 12 | 18 | 16 | 58 |
| Falak | 15 | 7 | 10 | 47 |
| Geeta | 14 | 0-20 | 6 | 34-54 |
| Harini | 5 | 0-20 | 0-20 | 50 |
| Indu | 0-20 | 8 | 0-20 | 8-68 |
| Jatin | 0-20 | 16 | 14 | 30-70 |
Point 2 states that Ajay is the unique high scorer in WE that means he scored the highest marks and no one had same marks as him in WE. As the highest score among other is 18, Ajay must have scored 19 or 20 and no one else could have scored 20 (they might score 19 if Ajay’s score is 20).
Point 3 and 4 says that 4 candidates were recruited including Geeta and Indu while Geeta was ranked 4th among these.
Point 6 tells us that Jatin and Indu, both scored a 20 in exactly 1 section and Jatin had higher composite score than Indu by 10. That means Jatin is among the 4 recruited too. And Jatin must have scored 20 in DI.
Also, Candidates who scored less than 70% in two or more sections are disqualified.
Using these in the table
| Candidate | DI *2 | WE | GA | Composite |
| Ajay | 8 | 19-20 | 16 | 51-52 |
| Bala (Dis) | 0-20 | 9 | 11 | 20-60 |
| Chetna (Dis) | 19 | 4 | 12 | 54 |
| Danish | 8 | 15 | 0-20 | 31-51 |
| Ester | 12 | 18 | 16 | 58 |
| Falak (Dis) | 15 | 7 | 10 | 47 |
| Geeta (Rec) | 14 | 0-19 | 6 | 34-53 |
| Harini | 5 | 0-19 | 0-20 | 49 |
| Indu (Rec) | 0-20 | 8 | 0-20 | 8-67 |
| Jatin (Rec) | 20 | 16 | 14 | 70 |
Rec means recruited and Dis means disqualified.
Jatin’s Composite score is 70 so Indu composite score must be 60 as her score was 10 less than Jatin.
This can be done in two ways, 1) if she score 16 in DI and 20 in GA, 2) if she score 20 in DI and 12 in GA. But in the second case, we see that Indu is scoring less that 70% in exactly 2 subjects which will disqualify her so this must be invalid case.
We also eliminate the case where some one else was scoring same score in Compoosite score as per point 1. That is for Bala. He cannot score 60 and 58 so his highest possible score must be 56.
| Candidate | DI *2 | WE | GA | Composite |
| Ajay | 8 | 19-20 | 16 | 51-52 |
| Bala (Dis) | 0-18 | 9 | 11 | 20-56 |
| Chetna (Dis) | 19 | 4 | 12 | 54 |
| Danish | 8 | 15 | 0-20 | 31-51 |
| Ester | 12 | 18 | 16 | 58 |
| Falak (Dis) | 15 | 7 | 10 | 47 |
| Geeta (Rec) | 14 | 0-19 | 6 | 34-53 |
| Harini | 5 | 0-19 | 0-20 | 49 |
| Indu (Rec) | 16 | 8 | 20 | 60 |
| Jatin (Rec) | 20 | 16 | 14 | 70 |
Point 5 tells us that Danish, Harini and Indu scored same marks in GA. That would make the table as:
| Candidate | DI *2 | WE | GA | Composite |
| Ajay | 8 | 19-20 | 16 | 51-52 |
| Bala (Dis) | 0-18 | 9 | 11 | 20-56 |
| Chetna (Dis) | 19 | 4 | 12 | 54 |
| Danish | 8 | 15 | 20 | 51 |
| Ester | 12 | 18 | 16 | 58 |
| Falak (Dis) | 15 | 7 | 10 | 47 |
| Geeta (Rec) | 14 | 0-19 | 6 | 34-53 |
| Harini | 5 | 0-19 | 20 | 30-49 |
| Indu (Rec) | 16 | 8 | 20 | 60 |
| Jatin (Rec) | 20 | 16 | 14 | 70 |
Now, we see that Danish is scoring 51 marks. But as point 1 says that no two people had same composite score, Ajay must have scored 52 only with 20 in WE.
In the table, among those who have not been rejected or recruited, Ester has the highest score and his sectional scores are also above 70% in 2 sections. Ester must be the 4th person recruited.
We know that Ajay and Danish are qualified candidates but their composite score is not in top 4 while Geeta’s score is so Geeta must have got 53 as her composite score.
Our table looks like this:
| Candidate | DI *2 | WE | GA | Composite |
| Ajay | 8 | 20 | 16 | 52 |
| Bala (Dis) | 0-18 | 9 | 11 | 20-56 |
| Chetna (Dis) | 19 | 4 | 12 | 54 |
| Danish | 8 | 15 | 20 | 51 |
| Ester (Rec) | 12 | 18 | 16 | 58 |
| Falak (Dis) | 15 | 7 | 10 | 47 |
| Geeta (Rec) | 14 | 19 | 6 | 53 |
| Harini | 5 | 0-19 | 20 | 30-49 |
| Indu (Rec) | 16 | 8 | 20 | 60 |
| Jatin (Rec) | 20 | 16 | 14 | 70 |
Question 1
Which of the following statements MUST be true?
1. Jatin’s composite score was more than that of Danish.
2. Indu scored less than Chetna in DI.
3. Jatin scored more than Indu in GA.
1. Both 1 and 2
2. Both 2 and 3
3. Only 2
4. Only 1
Answer: statement 1 says that Jatin scored more in composite score than Danish. As per the table, Danish scored 51 and Jatin scored 70 so this statement is true.
Statement 2 says that Indu scored less than Chetna in DI. As per the table, Chetna scored 19 in DI whereas Indu scored 16, so this is false.
Statement 3 says that Jatin scored more than Indu in GA. As per the table, Jatin scored 14 in GA which is less than Indu’s score of 20 so this is false.
Only statement 1 must be true hence option 4.
Question 2
Which of the following statements MUST be FALSE?
1. Bala’s composite score was less than that of Ester
2. Chetna scored more than Bala in DI
3. Harini’s composite score was less than that of Falak
4. Bala scored same as Jatin in DI
Answer: Statement 1 says that Bala’s composite score was less than that of ester. In the table, we can see that Bala can score up to 56 where as Ester scored 58. So this must be true.
Statement 2 says that Chetna scored more than Bala in DI. Chetna scored 19 in DI whereas highest possible score for Bala in DI is 18 so this is also true.
Statement 3 says that Harini’s composite score was less than that of Falak. Harini’s composite score can be anywhere among 30-49 where as Falak’s score is 47. This means that the statement can be true.
Statement 4 says that Bala scored same as Jatin in DI. As we know that Jatin scored 20 in DI where as Bala can only score 0 to 18 in DI hence this statement must be false.
Option 4 is the answer.
Question 3
If all the candidates except Ajay and Danish had different marks in DI, and Bala’s composite score was less than Chetna’s composite score, then what is the maximum mark that Bala could have scored in DI?
Answer: We include two points given in this question
- Everyone except Ajay and Danish scored different marks in DI. This means that Bala cannot score 5, 8, 12, 14, 15 and 16 either.
- Bala’s composite score was less than Chetna’s composite score. This implies that Bala cannon score 17 and 18 in DI, both because at 18, he scores more and at 17, he scores same Chetna.
Now, Bala can only score 13 in DI as 14 to 20 marks has been eliminated from his possible scores of DI. At 13, his composite score is 13 * 2 + 9 + 11 = 46 which is possible as nobody else have scored 46 yet.
So the answer would be 13.
Question 4
If all the candidates scored different marks in WE then what is the maximum marks that Harini could have scored in WE?
Answer: The given condition is that Harini can only score marks in WE that hasn’t been scored by anyone else. This eliminates 18 and 19.
At a score of 17 in WE, Harini’s composite score will be 5 * 2 + 17 + 20 =47.
As we see in the table, Falak’s score is also 47 so this cannot be Harini’s composite score.
Then 15 and 16 are also eliminated as they have been scored by others.
At a score of 14 in WE, Harini’s composite score will be 5 * 2 + 14 + 20 = 44, which hasn’t been scored by anyone else so this is possible.
14 is the answer for this question.

